Hello guys!
Most of you know about the legendary one stop CP Topic List created by YouKn0wWho (for those who don't know can check it out here).
Personally I'm not a fan of this cool UI in CP related things. I like it to be simple and it was too complex for my cyan brain to understand. (really personal opinion so don't jump on me for that :D)
So what i did is i scraped the ultimate topic list and put it all in a spreadsheet for me and y'all to have it.
Here's the link: CP Topic List (Jus make a copy and you'll be able to edit it as your personal sheet)
Here's an SS of how my personal one looks like :P
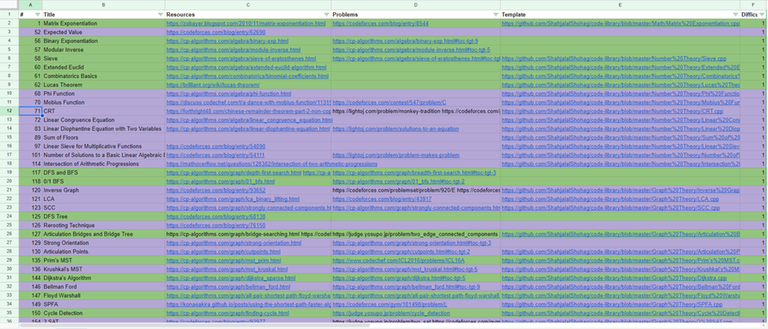
There's two things I'd also like you to know about this list.
You can sort everything by Serial Number and it will be divided chunk wise into more general topics like maths and graphs
You can sort everything by Difficulty and it will be divided into 3 chunks of increasing or decreasing difficulty.
I'd also like to ask you guys. what rating range does difficulty 1, 2 and 3 map to? feel free to comment and I'll attach the best ones in the blog for others.
Rating Ranges for Difficulties
- 1 — [0 — 2400] Quoting galen_colin, "you basically could get to red with just the basics every red coder in the forum agreed"
- 2 — [yet to be decided]
- 3 — [yet to be decided]
Thankyou for reading!
Special Thanks to ORZ YouKn0wWho