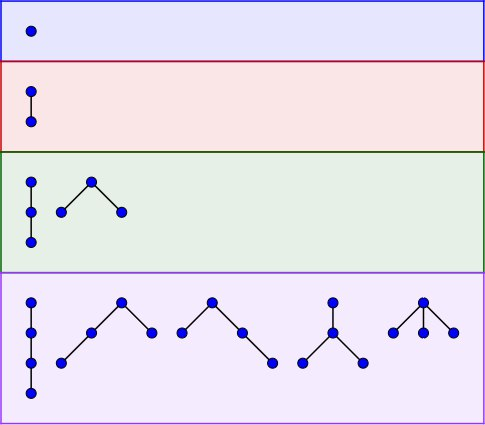
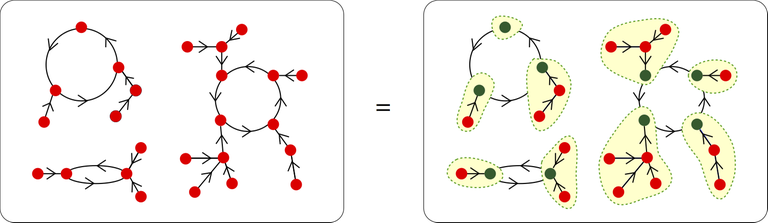
Hi everyone!
Some time ago the following "simple math problem" was shared in a Discord chat:
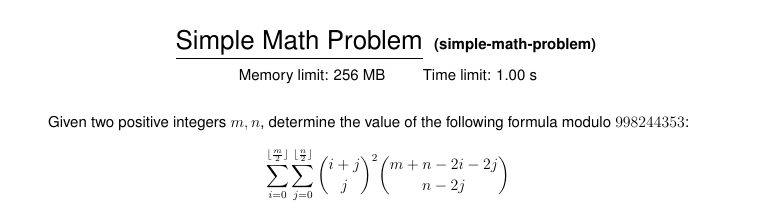
As a lover of simple math problems, I couldn't just pass by this problem. It turned out much harder than any other genfunc problem that I solved before, as the structure of the answer depends on the parity of $$$n$$$ and $$$m$$$, and it's not very natural to track it through genfuncs. It took me few months, I even called for help from higher powers (i.e. sent a pm to Elegia) but I finally have a solution that I somewhat like.