Origin URL (for Chinese): https://blog.csdn.net/can919/article/details/81840870
Translate by Baidu:
Subject matter
A tree with n nodes has two weights a and B on each edge. It takes time ai*t + b to pass through the first edge on day t. Given m, the longest path length can be obtained when t = 0, 1, 2... m-1.
An explanation
Briefly introduce Centroid decomposition on edges
Similar to Centroid decomposition on points, while dividing and conquering to select an edge, the tree is divided into two sides, so that the points on both sides are the closest.
However, edge partition and conquering of ordinary trees is easy to degenerate. The following graph will degenerate to O (n) (the official solution called it star tree) 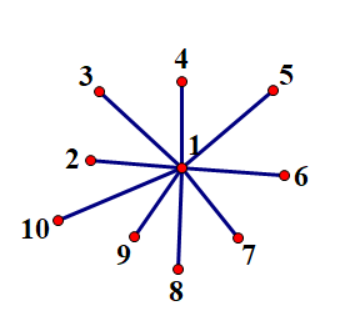
So using edge divide and conquer, we need to transform the general tree into a binary tree, so the edge divide and conquer is divided into two pieces, which ensures that the number of nodes between the two pieces must be between [n/3, n/2].
The advantage of Centroid decomposition on edges over Centroid decomposition on points is that it only divides the tree into two pieces, which in some cases reduces the amount of code needed to merge the results.
How to Centroid decomposition on edges in this problem
For this problem, we must ensure that the distance between any two points remains unchanged. When turning a binary tree, we need to create a new node, as follows: 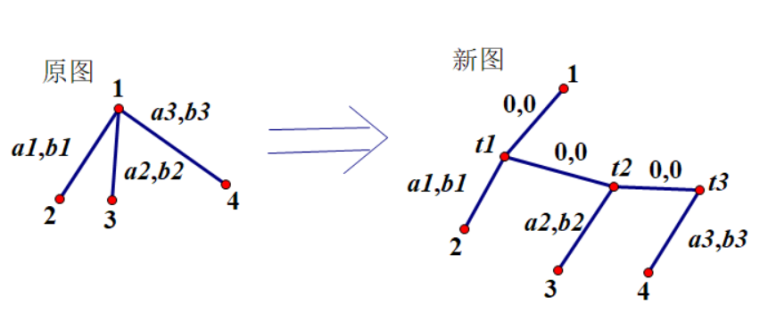 For the number of edge divide and conquer trees, calculate the size of each subtree siz [u], find the largest subtree and size less than half of the total size, select this node and its father node edge as the center of gravity edge, divided into two trees.
For the number of edge divide and conquer trees, calculate the size of each subtree siz [u], find the largest subtree and size less than half of the total size, select this node and its father node edge as the center of gravity edge, divided into two trees.
For the two trees
It is easy to know that the longest path must be from the root to the leaf node path, statistics of all paths, the value of a and B added up separately, get the function of this path a*t + b.
These two trees, we can get a bunch of paths function, merge two paths that add their a value and b value respectively, now consider how O (n) O (n) merge all paths.
Set ai<aj
ai*t+bi<aj*t+bj
−(bj−bi)<(aj−ai)t
(bj−bi)/(aj−ai)>−t
For each function at + B at + b, consider it as a point (a, b) (a, b), maintain the convex hull according to the slope formula, and decrease the slope (do not understand the visible slope optimization)
All the path functions of the two trees are divided into two convex hull respectively.
When merging, two variables x, y are used to represent the number of nodes currently merged into the two convex hulls. Each time the slope of X and x+1 : k1, y and y+1 : K2 are judged, the larger slope + 1 is selected, and then the new x node and Y node are merged (the merging of the larger slope ensures the smaller slope change in the new convex hull, so that the convex hull can be made. Middle nodes are not missed.)
After doing edge divide and conquer, we get the convex hull of all useful paths of the whole tree, which has guaranteed the monotony of the answer and can be calculated directly.
Code:
#include<cstdio>
#include<cstring>
#include<vector>
#include<algorithm>
using namespace std;
const int MAXN=200005,MAXM=MAXN*3;
struct Edge
{
int v,a,b,id;
Edge()=default;
Edge(int _v,int _a,int _b,int _id):v(_v),a(_a),b(_b),id(_id) {}
};
class Tree
{
public:
int n;
vector<Edge> adj[MAXN*2];
vector<Edge> &operator [] (unsigned int i)
{
return adj[i];
}
void AddEdge(int u,int v,int a,int b,int i)
{
adj[u].emplace_back(v,a,b,i);
adj[v].emplace_back(u,a,b,i);
}
};
struct Path
{
long long a,b;
Path()=default;
Path(long long _a,long long _b):a(_a),b(_b) {}
bool operator < (const Path &t)const
{
return a<t.a||(a==t.a&&b<t.b);
}
};
int N,M,edgeid;
Tree F,G;
void ToBinaryTree(int u,int fa=0)
{
int last=u;
for(const auto &e:F[u])
if(e.v!=fa)
{
G.AddEdge(last,++G.n,0,0,++edgeid);//fprintf(stderr,"[%d->%d: 0,0]\n",last,G.n);
G.AddEdge(G.n,e.v,e.a,e.b,++edgeid);//fprintf(stderr,"[%d->%d: %d,%d]\n",G.n,e.v,e.a,e.b);
last=G.n;
ToBinaryTree(e.v,u);
}
}
bool disable[MAXM];
int siz[MAXN];
Edge E[MAXN];
void GetSize(int u,int fa=0)
{
siz[u]=1;
for(const auto &e:G[u])
if(e.v!=fa&&!disable[e.id])
{
E[e.v]=Edge(u,e.a,e.b,e.id);
GetSize(e.v,u);
siz[u]+=siz[e.v];
}
}
int FindCentroid(int u,int lim,int fa=0)
{
if(siz[u]<=lim)
return u;
int mxsiz=0,id;
for(const auto &e:G[u])
if(e.v!=fa&&!disable[e.id])
{
int tmp=FindCentroid(e.v,lim,u);
if(siz[tmp]>mxsiz)
mxsiz=siz[tmp],id=tmp;
}
return id;
}
void GetPath(int u,vector<Path> &P,Path now=Path(0,0),int fa=0)
{
if(G[u].size()==1)
P.push_back(now);
for(const auto &e:G[u])
if(e.v!=fa&&!disable[e.id])
GetPath(e.v,P,Path(now.a+e.a,now.b+e.b),u);
}
void Process(vector<Path> &P)
{
static vector<Path> tmp;
sort(P.begin(),P.end());
tmp.resize(P.size());
tmp.emplace_back(0,0);
int top=0;
for(const auto &p:P)
{
while(top>0&&(p.a==tmp[top].a&&p.b>=tmp[top].b))
top--;
if(p.a==tmp[top].a&&p.b<=tmp[top].b)
continue;
while(top>0&&1.0*(tmp[top].b-tmp[top-1].b)/(tmp[top].a-tmp[top-1].a)<1.0*(p.b-tmp[top].b)/(p.a-tmp[top].a))
top--;
tmp[++top]=p;
}
for(int i=0; i<=top; i++)
P[i]=tmp[i];
P.resize(top+1);
}
vector<Path> P1,P2,P;
void CentroidDecomposition(int u)
{
GetSize(u);
if(siz[u]<=1)
return;
int centroid1=FindCentroid(u,siz[u]/2);
int centroid2=E[centroid1].v;
disable[E[centroid1].id]=true;
P1.clear();
P2.clear();
P1.emplace_back(0,0);
P2.emplace_back(0,0);
GetPath(centroid1,P1);
GetPath(centroid2,P2);
Process(P1);
Process(P2);
int x=0,y=0;
while(x<(int)P1.size()&&y<(int)P2.size())
{
P.emplace_back(P1[x].a+P2[y].a+E[centroid1].a,P1[x].b+P2[y].b+E[centroid1].b);
double k1=-1e100,k2=-1e100;
if(x<(int)P1.size()-1)
k1=1.0*(P1[x+1].b-P1[x].b)/(P1[x+1].a-P1[x].a);
if(y<(int)P2.size()-1)
k2=1.0*(P2[y+1].b-P2[y].b)/(P2[y+1].a-P2[y].a);
if(k1>k2)
x++;
else
y++;
}
CentroidDecomposition(centroid1);
CentroidDecomposition(centroid2);
}
int main()
{
scanf("%d%d",&N,&M);
F.n=N;
for(int i=1; i<N; i++)
{
int u,v,a,b;
scanf("%d%d%d%d",&u,&v,&a,&b);
F.AddEdge(u,v,a,b,i);
}
G.n=N;
ToBinaryTree(1);
P.emplace_back(0,0);
CentroidDecomposition(1);
Process(P);
int id=0;
for(int t=0; t<M; t++)
{
while(id<(int)P.size()-1&&(1LL*t*P[id+1].a+P[id+1].b)>(1LL*t*P[id].a+P[id].b))
id++;
long long ans=1LL*t*P[id].a+P[id].b;
printf("%I64d ",ans);
}
puts("");
return 0;
}










