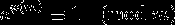Hi, I am having problem with solving this task from my math homework. If number p is prime and p^2+8 is also prime. Prove that p^3+4 is also prime number.
→ Pay attention
→ Top rated
| # | User | Rating |
|---|---|---|
| 1 | tourist | 4009 |
| 2 | jiangly | 3823 |
| 3 | Benq | 3738 |
| 4 | Radewoosh | 3633 |
| 5 | jqdai0815 | 3620 |
| 6 | orzdevinwang | 3529 |
| 7 | ecnerwala | 3446 |
| 8 | Um_nik | 3396 |
| 9 | ksun48 | 3390 |
| 10 | gamegame | 3386 |
→ Top contributors
| # | User | Contrib. |
|---|---|---|
| 1 | cry | 166 |
| 2 | maomao90 | 163 |
| 2 | Um_nik | 163 |
| 4 | atcoder_official | 161 |
| 5 | adamant | 160 |
| 6 | -is-this-fft- | 158 |
| 7 | awoo | 157 |
| 8 | TheScrasse | 154 |
| 9 | nor | 153 |
| 9 | Dominater069 | 153 |
→ Find user
→ Recent actions
Codeforces (c) Copyright 2010-2024 Mike Mirzayanov
The only programming contests Web 2.0 platform
Server time: Nov/17/2024 20:32:29 (i1).
Desktop version, switch to mobile version.
Supported by
User lists


| Name |
|---|











Suppose that p ≠ 3. Then thus p2 + 8 is not prime and we were wrong in our assumption. Then p = 3 and p3 + 4 = 31 which is obviously prime.
thus p2 + 8 is not prime and we were wrong in our assumption. Then p = 3 and p3 + 4 = 31 which is obviously prime.
How to prove that p2 = 1 mod 3?
Fermat's little theorem. If p is prime, then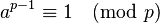
This is a special case of Euler's theorem, which is formulated like this for coprime a, m: