Hi!↵
↵
There's a problem I'd appreciate some help with (maybe hints or a sketch of solution...)↵
↵
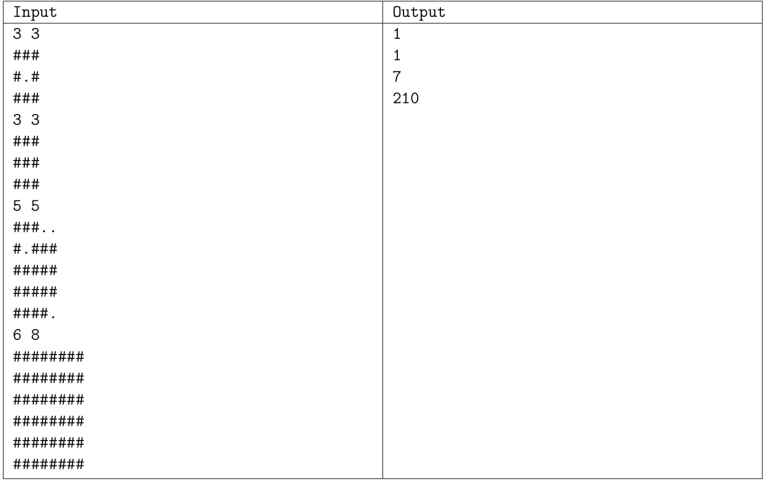
You have an `n \times n` grid, with `n \le 350`. Every element of the grid is either '.' or '#'.↵
↵
The problem asks to count the number of rectangles made of '#', where sides are bigger than or equal to 3.↵
↵
So the minimal rectangle would be↵
↵
~~~~~↵
###↵
#.#↵
###↵
~~~~~↵
↵
**IMPORTANT: There are 100 test cases, so the solution should be around O(N^2 lg N)**↵
↵
I tried to count the complement: As the total number of rectangles in a grid is easy to compute, I tried to focus on every dot and count the number of rectangles it interrupted, but I haven't figured out exactly how to avoid counting things multiple times...↵
↵
↵
There's a problem I'd appreciate some help with (maybe hints or a sketch of solution...)↵
↵
You have an `n \times n` grid, with `n \le 350`. Every element of the grid is either '.' or '#'.↵
↵
The problem asks to count the number of rectangles made of '#', where sides are bigger than or equal to 3.↵
↵
So the minimal rectangle would be↵
↵
~~~~~↵
###↵
#.#↵
###↵
~~~~~↵
↵
**IMPORTANT: There are 100 test cases, so the solution should be around O(N^2 lg N)**↵
↵
I tried to count the complement: As the total number of rectangles in a grid is easy to compute, I tried to focus on every dot and count the number of rectangles it interrupted, but I haven't figured out exactly how to avoid counting things multiple times...↵
↵






