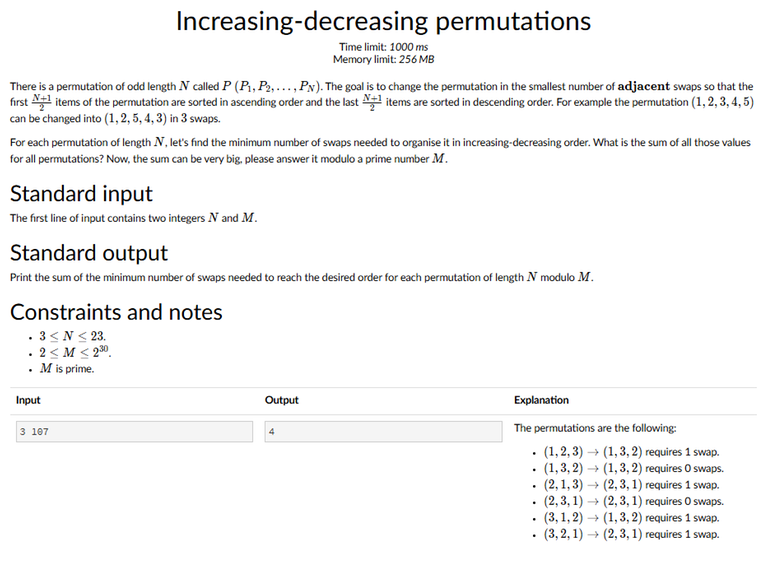
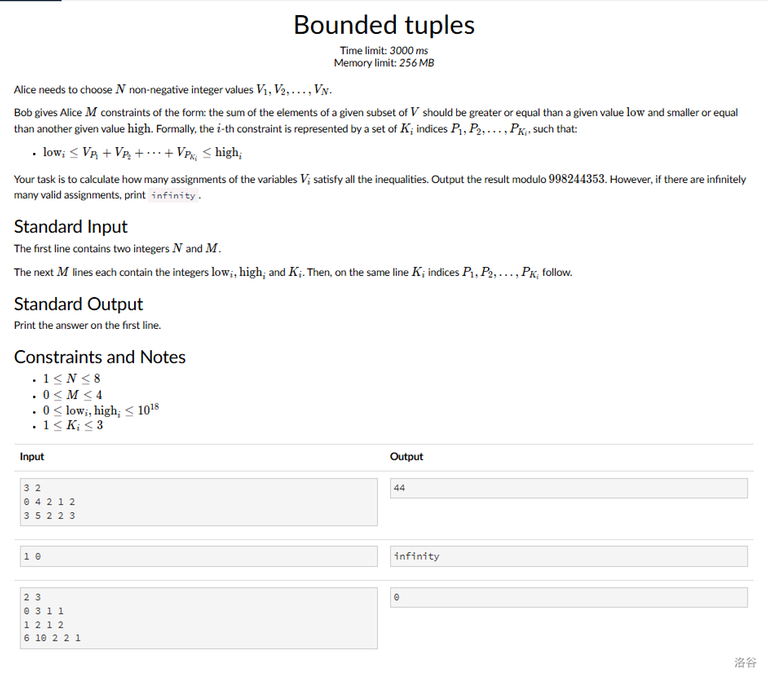
→ Pay attention
→ Top rated
| # | User | Rating |
|---|---|---|
| 1 | tourist | 3856 |
| 2 | jiangly | 3747 |
| 3 | orzdevinwang | 3706 |
| 4 | jqdai0815 | 3682 |
| 5 | ksun48 | 3591 |
| 6 | gamegame | 3477 |
| 7 | Benq | 3468 |
| 8 | Radewoosh | 3462 |
| 9 | ecnerwala | 3451 |
| 10 | heuristica | 3431 |
→ Top contributors
| # | User | Contrib. |
|---|---|---|
| 1 | cry | 167 |
| 2 | -is-this-fft- | 162 |
| 3 | Dominater069 | 160 |
| 4 | Um_nik | 158 |
| 5 | atcoder_official | 157 |
| 6 | Qingyu | 156 |
| 7 | adamant | 151 |
| 7 | djm03178 | 151 |
| 9 | luogu_official | 150 |
| 10 | awoo | 146 |
→ Find user
→ Recent actions
There are $$$n$$$ points on the line, the position of $$$i$$$ th point is $$$p[i]$$$. there are Q querys. you will got a segment which length is $$$D[i]$$$, ask at least how many segments are needed to cover all points.
$$$ 1 \le n \le 10^5 $$$, $$$ 1 \le p[i] \le 10^9 $$$, $$$ 1 \le q \le 10^5 $$$
n points on 2D-plane. $$$n \ge 3$$$.
Exist three points a,b,c that $$$1 \le \frac{dis(a,b)}{dis(a, c)} \le \frac{n+1}{n-1}$$$.
How to prove that?
Codeforces (c) Copyright 2010-2025 Mike Mirzayanov
The only programming contests Web 2.0 platform
Server time: Feb/23/2025 09:36:53 (j1).
Desktop version, switch to mobile version.
Supported by
User lists


| Name |
|---|