Hello.
I am trying to prove that sin (α - β) = sin α * cos β - cos α * sin β but getting something wrong.
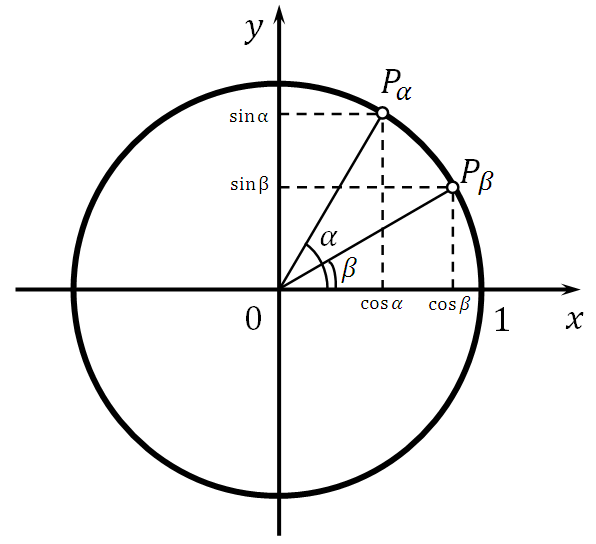
OPα = (cos α, sin α)
OPβ = (cos β, sin β)
|OPα × OPβ| = |OPα| * |OPβ| * sin (γ)
|OPα| = |OPβ| = 1
γ = α - β
Hence, |OPα × OPβ| = sin (α - β)
Since, |A × B| = Ax * By - Ay * Bx
|OPα × OPβ| = cos α * sin β - sin α * cos β
Hence, sin (α - β) = cos α * sin β - sin α * cos β
But all formulas in internet say that sin (α - β) = sin α * cos β - cos α * sin β , i.e reverse of what I wrote above. Can someone find my mistake?
P.S Below is the correct proof written in white. You can find my mistake yourself first. ;)
As I understood I made mistake in computing angle. Since angles are + in counter-clockwise direction then
|OPα × OPβ| = ... * sin(360 - γ)
|OPα × OPβ| = |OPα| * |OPβ| * ( - sin (γ))
Hence, |OPα × OPβ| = - sin (α - β)
And - sin (α - β) = cos α * sin β - sin α * cos β
Leads to sin (α - β) = sin α * cos β - cos α * sin β











You take absolute value only from part of the expression. I think it should be:
|OPα × OPβ| = |OPα| * |OPβ| * |sin (γ)|
|OPα × OPβ| = |sin (α - β)|
And as result:
|sin (α - β)| = |cos α * sin β - sin α * cos β|
And that is correct.
|A| is magnitude of vector A, not an absolute value. :D
as you know, A × B = - B × A, and you made such a mistake in order of operands in following expression: |OPα × OPβ| = sin(α - β).