Death_Scythe's blog

Finding sum of product of maximum and minimum over all subarrays
By Death_Scythe, history, 7 years ago, In English

Hi! I have been trying the following problem for some time now and haven't been able to come up with a solution better than O(N^2).

Given an array A with N integers, define maxi, j to be the maximum value in A[i..j] and mini, j to be the minimum value in A[i..j]. A[i..j] denotes the subarray starting from index i and ending at j. Compute the following sum:

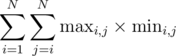
Please let me know if this problem is available on some OJ.

  • Vote: I like it
  • +20
  • Vote: I do not like it

Comments Comments (8)
| Write comment?
»
7 years ago, # |
  Vote: I like it +13 Vote: I do not like it

Your problem kinda looks like this: https://csacademy.com/contest/round-35/task/min-max-sum/statement/

→ Reply
  • »
    »
    7 years ago, # ^ |
      Vote: I like it +5 Vote: I do not like it

    I guess the editorial solution should work with my problem. I have to sum Vi without the pi - 1 factor.

    Thanks for the help!

    → Reply
    • »
      »
      »
      7 years ago, # ^ |
        Vote: I like it +5 Vote: I do not like it

      there is 1 more solution with divide and conquer, for a range [l, r], fix mid = (l + r) / 2 and find sum of max(i,j) * min(i, j) for ranges (i, j) that contain mid in O(r — l + 1)

      then u remove mid and solve for (l, mid — 1) and (mid + 1, r)

      → Reply
      • »
        »
        »
        »
        7 years ago, # ^ |
          Vote: I like it 0 Vote: I do not like it

        I did consider this way of thinking but I do not see how to compute the answer for ranges containing mid in linear time.

        → Reply
        • »
          »
          »
          »
          »
          7 years ago, # ^ |
            Vote: I like it +8 Vote: I do not like it

          range [i, j] containing mid will be a suffix [i, mid] and prefix [mid + 1, j]

          now you have to precalculate all suffix maxima , minima and same for prefix

          And you have 4 cases: minimum is in suffix, but maximum is in prefix

          or vice versa

          Or Both are in prefix, or Both are in suffix

          you need to treat all 4 cases separately

          note that suffix maxima is increasing, suffix minima is decreasing, prefix maxima is increasing, prefix minima is decreasing

          Now think how you can solve this with 2 pointers approach

          → Reply
»
7 years ago, # |
  Vote: I like it 0 Vote: I do not like it

I think that it can be solved using divide and conguer. The complexity will be O(nlogn)

→ Reply
»
7 years ago, # |
  Vote: I like it +3 Vote: I do not like it

Another very similar problem is Norma from COCI 2014/15.

You can submit it here.

→ Reply