How to solve this problem using matrix exponentiation. The recurrence relation is :
f(n, k, 0) = 2 * f(n - 1, k, 1) + f(n - 1, k, 0)
f(n, k, 1) = f(n - 1, k, 1) + f(n - 1, k, 0)
1 < n < 1e9
1 < k < 1e3
| № | Пользователь | Рейтинг |
|---|---|---|
| 1 | tourist | 3993 |
| 2 | jiangly | 3743 |
| 3 | orzdevinwang | 3707 |
| 4 | Radewoosh | 3627 |
| 5 | jqdai0815 | 3620 |
| 6 | Benq | 3564 |
| 7 | Kevin114514 | 3443 |
| 8 | ksun48 | 3434 |
| 9 | Rewinding | 3397 |
| 10 | Um_nik | 3396 |
| Страны | Города | Организации | Всё → |
| № | Пользователь | Вклад |
|---|---|---|
| 1 | cry | 167 |
| 2 | Um_nik | 163 |
| 3 | maomao90 | 162 |
| 3 | atcoder_official | 162 |
| 5 | adamant | 159 |
| 6 | -is-this-fft- | 158 |
| 7 | awoo | 157 |
| 8 | TheScrasse | 154 |
| 9 | Dominater069 | 153 |
| 9 | nor | 153 |
How to solve this problem using matrix exponentiation. The recurrence relation is :
f(n, k, 0) = 2 * f(n - 1, k, 1) + f(n - 1, k, 0)
f(n, k, 1) = f(n - 1, k, 1) + f(n - 1, k, 0)
1 < n < 1e9
1 < k < 1e3
| Название |
|---|



Matrix exponentiation is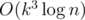 and it can be optimized to
and it can be optimized to 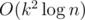 , but it's too slow and may still get TLE.
, but it's too slow and may still get TLE.
I used a O(k) solution.
Can you please explain your idea. I couldn't understand the editorial.
Can you provide a link to your solution or maybe tell your codechef handle so that I can look at your submission ? It will be helpful.
https://www.codechef.com/viewsolution/20839640
Thanks :)
The O(K) solution has been mentioned in the editorial.
I solved it by
Lagrange InterpolationLet f(n, k) denote the answer then we have the recurrence
Now $f(n, 1) = 2n $ , which is linear, which implies that f(n, 2) must be quadratic, which implies that f(n, 3) must be cubic and so on..
So for fixed k, f(n, k) will be a kth degree polynomial in n, and therefore i precomputed f(n, k) for n, k upto 2000 and then I answered each test case in O(n) using Lagrange Interpolation.
So overall time complexity O(K2 + TN)
My solution for reference
I have heard of this term first time. Maths is really very important for progress in CP. BTW thank you for your solution.
It can be done by combinatorics
https://www.codechef.com/viewsolution/20517309