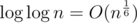Can anybodyt help to find complexity oof this algo: T(n) = n^ (1/3) T( sqrt( n) ) + 2n
I solved it and found it be O(n) but is it correct? Please confirm.
| # | User | Rating |
|---|---|---|
| 1 | jiangly | 4039 |
| 2 | tourist | 3841 |
| 3 | jqdai0815 | 3682 |
| 4 | ksun48 | 3590 |
| 5 | ecnerwala | 3542 |
| 6 | Benq | 3535 |
| 7 | orzdevinwang | 3526 |
| 8 | gamegame | 3477 |
| 9 | heuristica | 3357 |
| 10 | Radewoosh | 3355 |
| # | User | Contrib. |
|---|---|---|
| 1 | cry | 168 |
| 2 | -is-this-fft- | 165 |
| 3 | atcoder_official | 160 |
| 3 | Um_nik | 160 |
| 5 | djm03178 | 158 |
| 6 | Dominater069 | 156 |
| 7 | adamant | 153 |
| 8 | luogu_official | 152 |
| 9 | awoo | 151 |
| 10 | TheScrasse | 147 |
Can anybodyt help to find complexity oof this algo: T(n) = n^ (1/3) T( sqrt( n) ) + 2n
I solved it and found it be O(n) but is it correct? Please confirm.
| Name |
|---|



You can keep expanding T(n). By doing this, you get:
The first term is equal to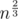 as n gets large, since the sum in the exponent is a geometric series.
as n gets large, since the sum in the exponent is a geometric series.
There are only log(log(n)) terms of the other kind. Proof: say that i is the last number for which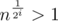 . Then
. Then 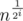 is approximately 2 (or 3, but if it is bigger we can take the root of that to get i+1), so we can take the logarithm from both sides of the equation
is approximately 2 (or 3, but if it is bigger we can take the root of that to get i+1), so we can take the logarithm from both sides of the equation 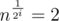 . This results in
. This results in 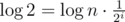 , so
, so 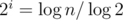 . Then we find for
. Then we find for 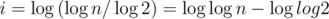
Every term of that kind have an exponent between 2/3 and 1. Say that we start counting these terms from i=0. Then the i'th term has an exponent equal to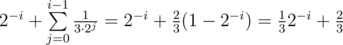 . So as you can see for i=0, this exponent is equal to 1, but as i gets larger, this exponent tends to 2/3.
. So as you can see for i=0, this exponent is equal to 1, but as i gets larger, this exponent tends to 2/3.
As n gets larger and larger, there are many more terms with exponents 2/3 than 1. So as n becomes large, the majority of the terms have an exponent which is approximately 2/3. Therefore the complexity of this algorithm is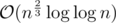
Shouldn't the upperbound be n as when exponent at i=0; exponent is 1. O( n + ( ( n^(2/3) ) * log( log(n) ) == O( n )?
Well, O(n) is a bit too small I guess. You could say that it is of order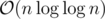 , because every term is smaller than 2n. I think the actual complexity of this algorithm is of the form
, because every term is smaller than 2n. I think the actual complexity of this algorithm is of the form 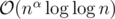 where α is a number between
where α is a number between  and 1.
and 1.
This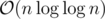 complexity differs from
complexity differs from 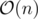 with a maximum factor of 6 for n smaller than 264, so you wouldn't see any difference for 'small' values of n. Still as n gets larger, this factor keeps increasing.
with a maximum factor of 6 for n smaller than 264, so you wouldn't see any difference for 'small' values of n. Still as n gets larger, this factor keeps increasing.
It's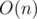 , because
, because 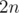 is
is 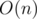 and each other summand is
and each other summand is 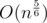 and there is
and there is 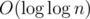 summands:
summands: 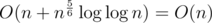 , because
, because