977D — Divide by three, multiply by two
We can see that all numbers in given sequence are distinct. since all numbers in sequence are of the form
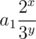 . Hence if ai = aj then
. Hence if ai = aj then 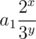 =
= 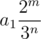

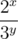 = \dfrac{2^m}{3^n}$ which is not possible because any power of 2 will be an even number and any power of 3 will be an odd number.
= \dfrac{2^m}{3^n}$ which is not possible because any power of 2 will be an even number and any power of 3 will be an odd number.if there exists
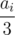 in sequence then 2$a_i$ can not be in sequence and vice versa. We can prove it using contradiction. Suppose there is a number ai such that both
in sequence then 2$a_i$ can not be in sequence and vice versa. We can prove it using contradiction. Suppose there is a number ai such that both Unable to parse markup [type=CF_TEX]
exists in sequence. by little bit of tricks this
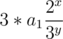 =
= 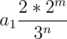 , this again is not possible by same argument as above, we just have to change the order of exponents.
, this again is not possible by same argument as above, we just have to change the order of exponents.Hence for each ai in sequence we see if
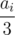 or 2$a_i$ is present(remember that only one of them can be present). Now if there is any ai such that both
or 2$a_i$ is present(remember that only one of them can be present). Now if there is any ai such that both 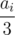 and 2$a_i$ is not in sequence then this shoudld be an. if there is any such ai that for all 0$\leq$ j \leq$
and 2$a_i$ is not in sequence then this shoudld be an. if there is any such ai that for all 0$\leq$ j \leq$  ≠ ai AND 2$a_i$ ≠ ai, then this a1.
≠ ai AND 2$a_i$ ≠ ai, then this a1.Once you have got a1 you keep on producing sequence just by doing binary search for
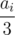 and 2$a_i$ (remember only one of them is present so once you find it you print it).
and 2$a_i$ (remember only one of them is present so once you find it you print it).






