I recently saw a question and was stuck on it for quite some time. Let's say if there are 'n' people standing is a circle. Now I will talk to the 1st person, then skip next 'a' people and talk to (a+2)-th person. I am supposed to calculate the number of people I have to talk to, before I can talk to the 1st person again.
Eg 1: The number of people = 10. The number of people to skip = 3.
so, the order in which I will go to people is -> 1 5 9 3 7 1 here, we can see that I had to go through 4 people to reach to 1 again.
Eg 2: The number of people = 10. The number of people to skip = 1
the order to reach out to people -> 1 3 5 7 9 1 here, 4 people were visited before reaching to 1 again.
Now the naive approach to solving this problem will be to iterate over the array but that will give me a time complexity of O(n) but if the number of people standing in the circle is of the order 10^18. This will not work.I think a better way to approach this problem would be by the help of gcd operation but I am not able to figure out the correct specifics of that approach. Also, Is there a approach to solve these type of questions.











Constraints on a? If it is less than 107, then there is an O(N) solution that would be fast enough.
Thanks for commenting, Rishi. Although that would be correct; I am looking for a solution which will be work in O(1) or at least in O (log n).
Isn't the first sequence wrong? 1, 5, 9, the next number should be 3. Coz we skip 10, 1 and 2.
Yes, It should be. I corrected it now. Thanks
If what I understood is correct, the answer to the problem is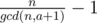
Here is the proof:
We need to visit 1 again. Let us suppose we visit it after going over the array [1, n], t times. To make it clear, t means how many times you skip the nth person.
So you need to solve this equation for x(can be obtained by analysing a simple AP):
t × n + 1 = 1 + (x - 1) × (a + 1).
Therefore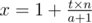 .
.
For this to be an integer, t should be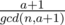 .
.
Here x will give us the xth term of A.P., so we need to subtract 2 because you want to find out how many terms you skip.
So substituting t and subtracting 2, we get
You might need to handle some corner cases, haven't given much thought.
Yes, This will work. I was just looking around gcd but was not able to figure out this particular expression. Thanks for the help :)
Auto comment: topic has been updated by abbi_18 (previous revision, new revision, compare).