Hey! I have a question. If I have to calculate a pow b modulo mod, with b>mod, is it the same with a pow (b % (mod-1)?
→ Pay attention
→ Top rated
| # | User | Rating |
|---|---|---|
| 1 | tourist | 3985 |
| 2 | jiangly | 3814 |
| 3 | jqdai0815 | 3682 |
| 4 | Benq | 3529 |
| 5 | orzdevinwang | 3526 |
| 6 | ksun48 | 3517 |
| 7 | Radewoosh | 3410 |
| 8 | hos.lyric | 3399 |
| 9 | ecnerwala | 3392 |
| 9 | Um_nik | 3392 |
→ Top contributors
| # | User | Contrib. |
|---|---|---|
| 1 | cry | 169 |
| 2 | maomao90 | 162 |
| 2 | Um_nik | 162 |
| 4 | atcoder_official | 161 |
| 5 | djm03178 | 158 |
| 6 | -is-this-fft- | 157 |
| 7 | adamant | 155 |
| 8 | awoo | 154 |
| 8 | Dominater069 | 154 |
| 10 | luogu_official | 150 |
→ Find user
→ Recent actions
↑
↓
Codeforces (c) Copyright 2010-2024 Mike Mirzayanov
The only programming contests Web 2.0 platform
Server time: Dec/22/2024 17:04:04 (i2).
Desktop version, switch to mobile version.
Supported by
User lists


| Name |
|---|











By Euler's Theorem, if gcd(a, n) = 1,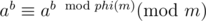 . If m happens to be a prime, then phi(m) = m - 1.
. If m happens to be a prime, then phi(m) = m - 1.
If "gcd(a,n)=1", you mean gcd(a,m)=1? I can not see the n
Upps, sorry. You're right, that was meant to be an m :)
Thanks!
Even when gcd(a, n) > 1, the following holds: If b ≥ φ(m),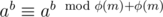 . When m is large and you cannot efficiently factor it to compute φ(m) and b is given in decimal form, you can still use fast exponentiation. Process digits of b one by one starting from the most significant one and use a10b + c = (ab)10 × ac.
. When m is large and you cannot efficiently factor it to compute φ(m) and b is given in decimal form, you can still use fast exponentiation. Process digits of b one by one starting from the most significant one and use a10b + c = (ab)10 × ac.